Wetting of solid surfaces and powders

Wetting of solid surfaces and powders

The wetting of a solid when a liquid spreads over its surface is referred to as spreading wetting.

• The forces acting on a drop on the solidsurface (Figure 1.4a) are represented by

Young’s equation:
γS/A = γS/L + γL/A cos θ

where γS/A is the surface tension of the
solid,
 γS/L is the solid–liquid interfacial tension,
 γL/A is the
surface tension of the liquid and θ is the contact angle.

• The tendency for wetting is expressed by the spreading

coefficient, S, as:
S = γL/A (cos θ – 1)

• For complete spreading of the liquid over the solid surface,S should have a zero or positive valve
•  If the contact angle is larger than 0°, the term (cos θ – 1) will be-ive, as will the value of S.
•  The condition for complete, spontaneous wetting is thus a zero valve the contact angle.
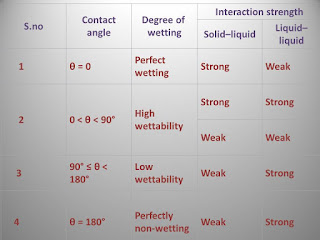
• The effectiveness of immersional wetting may be related to the contact angle which the solid makes with the liquid–air interface.
•  Contact angles of greater than 90° indicate wetting problems,for example when the drugs are formulated as suspensions.
•  Examples of very hydrophobic (non-wetting) drugs include magnesium and aluminium stearates, salicylic acid,phenylbutazone and chloramphenicol palmitate.
• The normal method of improving wettability is by the inclusion of surfactants in the formulation. The surfactants not only reduce γL/A but also adsorb on to the surface of the powder, thus reducing γS/L. Both of these effects reduce the contact angle and improve the dispersibility of the powder.